Image Processing with Deconvolution
Deconvolution is a computationally intensive image processing technique used to improve the contrast and sharpness of images captured using a light microscope. Light microscopes are diffraction limited, which means they are unable to resolve individual structures unless they are more than half the wavelength of light away from one another. Each point source below this diffraction limit is blurred by the microscope into what is known as a point spread function (PSF). With traditional widefield fluorescence microscopy, out-of-focus light from areas above or below the focal plane causes additional blurring in the captured image. Deconvolution removes or reverses this degradation by using the optical system’s point spread function and reconstructing an ideal image made from a collection of smaller point sources.
A light microscope’s point spread function varies based on the optical properties of both the microscope and the sample, making it difficult to experimentally determine the exact point spread function of the complete system. For this reason, mathematical algorithms have been developed to determine the point spread function and to make the best possible reconstruction of the ideal image using deconvolution. Nearly any image acquired with a fluorescence microscope can be deconvolved, including those that are not three dimensional.
Commercial software brings these algorithms together into cost-effective, user-friendly packages. Each deconvolution algorithm differs in how the point spread and noise functions of the convolution operations are determined. The basic imaging formula is:
g(x) = f(x) * h(x) + n(x)
x: Spatial coordinate
g(x): Observed image
f(x): Object
h(x): Point spread function
n(x): Noise function
*: Convolution
Deblurring Algorithms
Deblurring algorithms apply an operation to each two-dimensional plane of a three-dimensional image stack. A common deblurring technique, nearest neighbor, operates on each z-plane by blurring the neighboring planes (z + 1 and z - 1, using a digital blurring filter), then subtracting the blurred planes from the z-plane. Multi-neighbor techniques extend this concept to a user-selectable number of planes. A three-dimensional stack is processed by applying the algorithm to every plane in the stack.
This class of deblurring algorithms is computationally economical because it involves relatively simple calculations performed on a small number of image planes. However, there are several disadvantages to these approaches. For example, structures whose point spread functions overlap each other in nearby z-planes may be localized in planes where they do not belong, altering the apparent position of the object. This problem is particularly severe when deblurring a single two-dimensional image because it often contains diffraction rings or light from out-of-focus structures that will then be sharpened as if they were in the correct focal plane.
Inverse Filter Algorithms
An inverse filter functions by taking the Fourier transform of an image and dividing it by the Fourier transform of the point spread function. Division in Fourier space is equivalent to deconvolution in real space, making inverse filtering the simplest method to reverse the convolution in the image. The calculation is fast, with a similar speed as two-dimensional deblurring methods. However, the method’s utility is limited by noise amplification. During division in Fourier space, small noise variations in the Fourier transform are amplified by the division operation. The result is that blur removal is compromised as a tradeoff against a gain in noise. This technique can also introduce an artifact known as ringing.
Additional noise and ringing can be reduced by making some assumptions about the structure of the object that gave rise to the image. For instance, if the object is assumed to be relatively smooth, noisy solutions with rough edges can be eliminated. Regularization can be applied in one step within an inverse filter, or it can be applied iteratively. The result is an image stripped of high Fourier frequencies, resulting in a smoother appearance. Much of the "roughness" removed in the image resides at Fourier frequencies well beyond the resolution limit and, therefore, the process does not eliminate structures recorded by the microscope. However, because there is a potential for loss of detail, software implementations of inverse filters typically include an adjustable parameter that enables the user to control the tradeoff between smoothing and noise amplification. In most image-processing software programs, these algorithms have a variety of names including Wiener deconvolution, Regularized Least Squares, Linear Least Squares, and Tikhonov-Miller regularization.
Constrained Iterative Algorithms
A typical constrained iterative algorithm improves the performance of inverse filters by applying additional algorithms to restore photons to the correct position. These methods operate in successive cycles based on results from previous cycles, hence the term iterative. An initial estimate of the object is performed and convolved with the point spread function. The resulting "blurred estimate" is compared with the raw image to compute an error criterion that represents how similar the blurred estimate is to the raw image. Using the information contained in the error criterion, a new iteration takes place—the new estimate is convolved with the point spread function, a new error criterion is computed, and so on. The best estimate is the one that minimizes the error criterion. As the algorithm progresses, each time the software determines that the error criterion has not been minimized, a new estimate is blurred again, and the error criterion recomputed. The cycle is repeated until the error criterion is minimized or reaches a defined threshold. The final restored image is the object estimate at the last iteration.
The constrained iterative algorithms offer good results, but they are not suitable for all imaging setups. They require long calculation times and place a high demand on computer processors. This can be overcome with modern technologies, such as GPU-based processing which significantly improves speed. To take full advantage of the algorithms, three-dimensional images are required, though two-dimensional images can be used with limited performance.
Confocal, Multiphoton, and Super Resolution
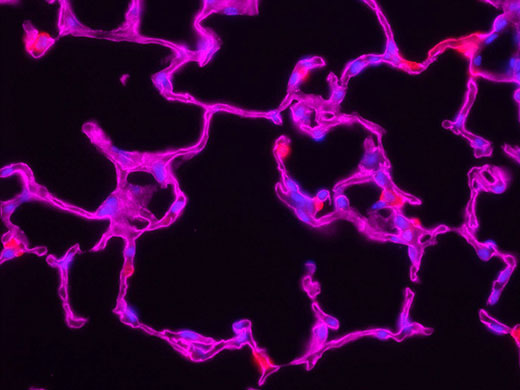
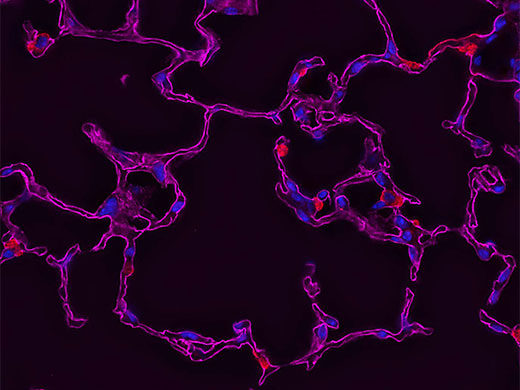
Some recommend deconvolution as an alternate technique to using a confocal microscope1. This is not strictly true because deconvolution techniques can also be applied to the images acquired using the pinhole aperture in a confocal microscope. In fact, it is possible to restore images acquired with a confocal, multiphoton, or super resolution light microscope.
The combination of optical image improvement through confocal or super resolution microscopy and deconvolution techniques improves sharpness beyond what is generally attainable with either technique alone. However, the major benefit of deconvolving images from these specialized microscopes is decreased noise in the final image. This is particularly helpful for low-light applications like live cell super resolution or confocal imaging. Deconvolution of multiphoton images has also been successfully utilized to remove noise and improve contrast. In all cases, care must be taken to apply an appropriate point spread function, especially if the confocal pinhole aperture is adjustable.
*1 Shaw, Peter J., and David J. Rawlins. “The point-spread function of a confocal microscope: its measurement and use in deconvolution of 3-D data.” Journal of Microscopy. 163, Issue no. 2 (1991): 151–165.
 |  |
Deconvolution in Practice
Processing speed and quality are dramatically affected by how software implements the deconvolution algorithm. The algorithm can be optimized to reduce the number of iterations and accelerate convergence to produce a stable estimate. For example, an unoptimized Jansson-Van Cittert algorithm usually requires between 50 and 100 iterations to converge to an optimal estimate. By prefiltering the raw image to suppress noise and correcting with an additional error criterion on the first two iterations, the algorithm converges in only 5 to 10 iterations.
When using an empirical point spread function, it is critical to use a high-quality point spread function with minimal noise. To achieve this, commercial software packages contain preprocessing routines that reduce noise and enforce radial symmetry by averaging the Fourier transform of the point spread function. Many software packages also enforce axial symmetry in the point spread function and assume the absence of spherical aberration. These steps reduce the empirical point spread function’s noise and aberrations and make a significant difference in the restoration’s quality.
Preprocessing can also be applied to raw images using routines such as background subtraction and flatfield correction. These operations can improve the signal-to-noise ratio and remove certain artifacts that are detrimental to the final image.
In general, the more faithful the data representation, the more computer memory and processor time are required to deconvolve an image. Previously, images would be divided into subvolumes to accommodate processing power, but modern technologies have reduced this barrier and expanded into larger data sets.
Olympus Deconvolution Solutions
Olympus’ cellSens imaging software features TruSight deconvolution, which combines commonly used deconvolution algorithms with new techniques designed for use on images acquired with Olympus FV3000 and SpinSR10 microscopes, delivering a full portfolio of tools for image processing and analysis.
Lauren Alvarenga
Scientific Solutions Group
Olympus Corporation of the Americas
Sorry, this page is not
available in your country.